Regular tree grammar

In theoretical computer science and formal language theory, a regular tree grammar (RTG) is a formal grammar that describes a set of directed trees, or terms.^[1] A regular word grammar can be seen as a special kind of regular tree grammar, describing a set of single-path trees.

Definition

A regular tree grammar G is defined by the tuple

G = (N, Σ, Z, P),

where

N is a finite set of nonterminals,
Σ is a ranked alphabet (i.e., an alphabet whose symbols have an associated arity) disjoint from N,
Z is the starting nonterminal, with Z ∈ N, and
P is a finite set of productions of the form A → t, with A ∈ N, and t ∈ T_Σ(N), where T_Σ(N) is the associated term algebra, i.e. the set of all trees composed from symbols in Σ ∪ N according to their arities, where nonterminals are considered nullary.

Derivation of trees

The grammar G implicitly defines a set of trees: any tree that can be derived from Z using the rule set P is said to be described by G. This set of trees is known as the language of G. More formally, the relation ⇒_G on the set T_Σ(N) is defined as follows:

A tree t₁∈ T_Σ(N) can be derived in a single step into a tree t₂ ∈ T_Σ(N) (in short: t₁ ⇒_G t₂), if there is a context S and a production (A→t) ∈ P such that:

t₁ = S[A], and
t₂ = S[t].

Here, a context means a tree with exactly one hole in it; if S is such a context, S[t] denotes the result of filling the tree t into the hole of S.

The tree language generated by G is the language L(G) = { t ∈ T_Σ | Z ⇒_G* t }.

Here, T_Σ denotes the set of all trees composed from symbols of Σ, while ⇒_G* denotes successive applications of ⇒_G.

A language generated by some regular tree grammar is called a regular tree language.

Examples

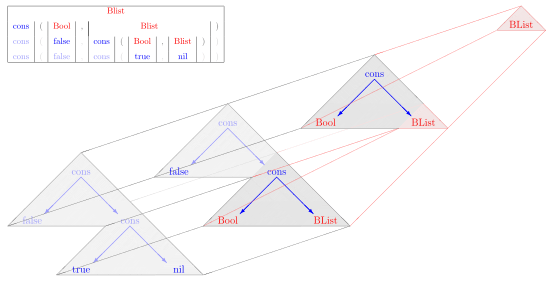
Example derivation tree from G₁ in linear (upper left table) and graphical (main picture) notation

Let G₁ = (N₁,Σ₁,Z₁,P₁), where

N₁ = {Bool, BList } is our set of nonterminals,
Σ₁ = { true, false, nil, cons(.,.) } is our ranked alphabet, arities indicated by dummy arguments (i.e. the symbol cons has arity 2),
Z₁ = BList is our starting nonterminal, and
the set P₁ consists of the following productions:
- Bool → false
- Bool → true
- BList → nil
- BList → cons(Bool,BList)

An example derivation from the grammar G₁ is

BList ⇒ cons(Bool,BList) ⇒ cons(false,cons(Bool,BList)) ⇒ cons(false,cons(true,nil)).

The image shows the corresponding derivation tree; it is a tree of trees (main picture), whereas a derivation tree in word grammars is a tree of strings (upper left table).

The tree language generated by G₁ is the set of all finite lists of boolean values, that is, L(G₁) happens to equal T_Σ1. The grammar G₁ corresponds to the algebraic data type declarations (in the Standard ML programming language):

  datatype Bool
    = false
    | true
  datatype BList
    = nil
    | cons of Bool * BList

Every member of L(G₁) corresponds to a Standard-ML value of type BList.

For another example, let G₂ = (N₁,Σ₁,BList1,P₁ ∪ P₂), using the nonterminal set and the alphabet from above, but extending the production set by P₂, consisting of the following productions:

BList1 → cons(true,BList)
BList1 → cons(false,BList1)

The language L(G₂) is the set of all finite lists of boolean values that contain true at least once. The set L(G₂) has no datatype counterpart in Standard ML, nor in any other functional language. It is a proper subset of L(G₁). The above example term happens to be in L(G₂), too, as the following derivation shows:

BList1 ⇒ cons(false,BList1) ⇒ cons(false,cons(true,BList)) ⇒ cons(false,cons(true,nil)).

Language properties

If L₁, L₂ both are regular tree languages, then the tree sets L₁ ∩ L₂, L₁ ∪ L₂, and L₁ \ L₂ are also regular tree languages, and it is decidable whether L₁ ⊆ L₂, and whether L₁ = L₂.

Alternative characterizations and relation to other formal languages

Rajeev Alur and Parthasarathy Madhusudan related a subclass of regular binary tree languages to nested words and visibly pushdown languages.^[2]^[3]

The regular tree languages are also the languages recognized by bottom-up tree automata and nondeterministic top-down tree automata.^[4]

Regular tree grammars are a generalization of regular word grammars.

Applications

Applications of regular tree grammars include:

Instruction selection in compiler code generation^[5]
A decision procedure for the first-order logic theory of formulas over equality (=) and set membership (∈) as the only predicates^[6]
Solving constraints about mathematical sets^[7]
The set of all truths expressible in first-order logic about a finite algebra (which is always a regular tree language)^[8]

References

↑ "Regular tree grammars as a formalism for scope underspecification". CiteSeerX 10.1.1.164.5484.
↑ Alur, R.; Madhusudan, P. (2004). "Visibly pushdown languages" (PDF). Proceedings of the thirty-sixth annual ACM symposium on Theory of computing - STOC '04. pp. 202–211. doi:10.1145/1007352.1007390. ISBN 1581138520. Sect.4, Theorem 5,
↑ Alur, R.; Madhusudan, P. (2009). "Adding nesting structure to words" (PDF). Journal of the ACM. 56 (3): 1–43. doi:10.1145/1516512.1516518. Sect.7
↑ Comon, Hubert; Dauchet, Max; Gilleron, Remi; Löding, Christof; Jacquemard, Florent; Lugiez, Denis; Tison, Sophie; Tommasi, Marc (12 October 2007). "Tree Automata Techniques and Applications". Retrieved 25 January 2016.
↑ Emmelmann, Helmut (1991). "Code Selection by Regularly Controlled Term Rewriting". Code Generation - Concepts, Tools, Techniques. Workshops in Computing. Springer. pp. 3–29.
↑ Comon, Hubert (1990). "Equational Formulas in Order-Sorted Algebras". Proc. ICALP.
↑ Gilleron, R.; Tison, S.; Tommasi, M. (1993). "Solving Systems of Set Constraints using Tree Automata". 10th Annual Symposium on Theoretical Aspects of Computer Science. LNCS. 665. Springer. pp. 505–514.
↑ Burghardt, Jochen (2002). "Axiomatization of Finite Algebras" (PDF). Advances in Artificial Intelligence. LNAI. 2479. Springer. pp. 222–234. ISBN 3-540-44185-9.

Regular tree grammars were already described in 1968 by:
- Brainerd, W.S. (1968). "The Minimalization of Tree Automata" (pdf). Information and Control. 13: 484–491. doi:10.1016/s0019-9958(68)90917-0.
- Thatcher, J.W.; Wright, J.B. (1968). "Generalized Finite Automata Theory with an Application to a Decision Problem of Second-Order Logic". Mathematical Systems Theory. 2 (1).
A book devoted to tree grammars is: Nivat, Maurice; Podelski, Andreas (1992). Tree Automata and Languages. Studies in Computer Science and Artificial Intelligence. 10. North-Holland.
Algorithms on regular tree grammars are discussed from an efficiency-oriented view in: Aiken, A.; Murphy, B. (1991). "Implementing Regular Tree Expressions". ACM Conference on Functional Programming Languages and Computer Architecture. pp. 427–447.
Given a mapping from trees to weights, Donald Knuth's generalization of Dijkstra's shortest-path algorithm can be applied to a regular tree grammar to compute for each nonterminal the minimum weight of a derivable tree. Based on this information, it is straightforward to enumerate its language in increasing weight order. In particular, any nonterminal with infinite minimum weight produces the empty language. See: Knuth, D.E. (1977). "A Generalization of Dijkstra's Algorithm". Information Processing Letters. 6 (1): 1–5. doi:10.1016/0020-0190(77)90002-3.
Regular tree automata have been generalized to admit equality tests between sibling nodes in trees. See: Bogaert, B.; Tison, Sophie (1992). "Equality and Disequality Constraints on Direct Subterms in Tree Automata". Proc. 9th STACS. LNCS. 577. Springer. pp. 161–172.
Allowing equality tests between deeper nodes leads to undecidability. See: Tommasi, M. (1991). Automates d'Arbres avec Tests d'Égalités entre Cousins Germains. LIFL-IT.

Automata theory: formal languages and formal grammars

Chomsky hierarchy	Grammars	Languages	Abstract machines

Type-0 — Type-1 — — — — — Type-2 — — Type-3 — —	Unrestricted (no common name) Context-sensitive Positive range concatenation Indexed — Linear context-free rewriting systems Tree-adjoining Context-free Deterministic context-free Visibly pushdown Regular — Non-recursive	Recursively enumerable Decidable Context-sensitive Positive range concatenation^* Indexed^* — Linear context-free rewriting language Tree-adjoining Context-free Deterministic context-free Visibly pushdown Regular Star-free Finite	Turing machine Decider Linear-bounded PTIME Turing Machine Nested stack Thread automaton restricted Tree stack automaton Embedded pushdown Nondeterministic pushdown Deterministic pushdown Visibly pushdown Finite Counter-free (with aperiodic finite monoid) Acyclic finite

Each category of languages, except those marked by a ^*, is a proper subset of the category directly above it. Any language in each category is generated by a grammar and by an automaton in the category in the same line.

This article is issued from Wikipedia - version of the 10/30/2016. The text is available under the Creative Commons Attribution/Share Alike but additional terms may apply for the media files.