Isolated point
In mathematics, a point x is called an isolated point of a subset S (in a topological space X) if x is an element of S but there exists a neighborhood of x which does not contain any other points of S. This is equivalent to saying that the singleton {x} is an open set in the topological space S (considered as a subspace of X). If the space X is a Euclidean space (or any other metric space), then x is an isolated point of S if there exists an open ball around x which contains no other points of S. (Introducing the notion of sequences and limits, one can say equivalently that a point x is an isolated point of S if and only if it is not a limit point of S.)
A set which is made up only of isolated points is called a discrete set. Any discrete subset S of Euclidean space must be countable, since the isolation of each of its points together with the fact the rationals are dense in the reals means that the points of S may be mapped into a set of points with rational coordinates, of which there are only countably many. However, not every countable set is discrete, of which the rational numbers under the usual Euclidean metric are the canonical example. See also discrete space.
A set with no isolated point is said to be dense-in-itself (every neighborhood of a point contains other points of the set). A closed set with no isolated point is called a perfect set (it has all its limit points and none of them are isolated from it).
The number of isolated points is a topological invariant, i.e. if two topological spaces and are homeomorphic, the number of isolated points in each is equal.
Standard Examples
Topological spaces in the following examples are considered as subspaces of the real line with the standard topology.
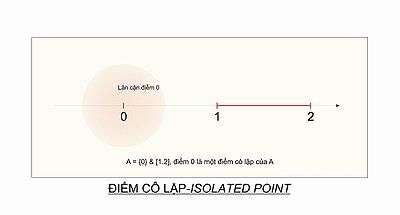
- For the set , the point 0 is an isolated point.
- For the set , each of the points 1/k is an isolated point, but 0 is not an isolated point because there are other points in S as close to 0 as desired.
- The set of natural numbers is a discrete set.
- The Morse lemma states that non-degenerate critical points of certain functions are isolated.
A Counter-intuitive Example
Let us consider the set of points in the real interval such that every digit of their binary representation fulfills the following conditions:
- Either or .
- only for finitely many indexes .
- If denotes the biggest index such that , then .
- If and , then exactly one of the following two condition holds: , . Informally, this condition means that every digit of the binary representation of equals to one, has a consecutive (digit-one) pair, but the last one.
Now, is an explicit set consisting entirely of isolated points.[1] Besides, has the counter-intuitive property that its closure is an uncountable set.[2]
Another set with the same properties can be obtained as follows. Let be the middle-thirds Cantor set, let be the component intervals of , and let be a set consisting of one point from each . Since each contains only one point from , every point of is an isolated point. However, if is any point in the Cantor set, then every neighborhood of contains at least one , and hence at least one point of . It follows that each point of the Cantor set lies in the closure of , and therefore has uncountable closure.
See also
References
- ↑ Gomez-Ramirez 2007, p.146-147
- ↑ Gomez-Ramirez 2007, p. 146
- Gomez-Ramirez, Danny (2007), "An explicit set of isolated points in R with uncountable closure", Matemáticas: Enseñanza universitaria, Escuela Regional de Matemáticas. Universidad del Valle, Colombia, 15: 145–147