Gegenbauer polynomials

In mathematics, Gegenbauer polynomials or ultraspherical polynomials C(α)
n(x) are orthogonal polynomials on the interval [−1,1] with respect to the weight function (1 − x²)^α–1/2. They generalize Legendre polynomials and Chebyshev polynomials, and are special cases of Jacobi polynomials. They are named after Leopold Gegenbauer.

Characterizations

Gegenbauer polynomials with α=1
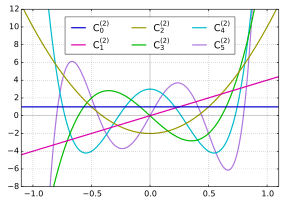
Gegenbauer polynomials with α=2
Gegenbauer polynomials with α=3
Gegenbauer polynomials

A variety of characterizations of the Gegenbauer polynomials are available.

The polynomials can be defined in terms of their generating function (Stein & Weiss 1971, §IV.2):

{\frac {1}{(1-2xt+t^{2})^{\alpha }}}=\sum _{{n=0}}^{\infty }C_{n}^{{(\alpha )}}(x)t^{n}.

The polynomials satisfy the recurrence relation (Suetin 2001):

{\begin{aligned}C_{0}^{\alpha }(x)&=1\\C_{1}^{\alpha }(x)&=2\alpha x\\C_{n}^{\alpha }(x)&={\frac {1}{n}}[2x(n+\alpha -1)C_{{n-1}}^{\alpha }(x)-(n+2\alpha -2)C_{{n-2}}^{\alpha }(x)].\end{aligned}}

Gegenbauer polynomials are particular solutions of the Gegenbauer differential equation (Suetin 2001):

(1-x^{{2}})y''-(2\alpha +1)xy'+n(n+2\alpha )y=0.\,

When α = 1/2, the equation reduces to the Legendre equation, and the Gegenbauer polynomials reduce to the Legendre polynomials.

They are given as Gaussian hypergeometric series in certain cases where the series is in fact finite:

C_{n}^{{(\alpha )}}(z)={\frac {(2\alpha )_{n}}{n!}}\,_{2}F_{1}\left(-n,2\alpha +n;\alpha +{\frac {1}{2}};{\frac {1-z}{2}}\right).

(Abramowitz & Stegun p. 561). Here (2α)_n is the rising factorial. Explicitly,

C_{n}^{{(\alpha )}}(z)=\sum _{{k=0}}^{{\lfloor n/2\rfloor }}(-1)^{k}{\frac {\Gamma (n-k+\alpha )}{\Gamma (\alpha )k!(n-2k)!}}(2z)^{{n-2k}}.

They are special cases of the Jacobi polynomials (Suetin 2001):

C_{n}^{{(\alpha )}}(x)={\frac {(2\alpha )_{n}}{(\alpha +{\frac {1}{2}})_{{n}}}}P_{n}^{{(\alpha -1/2,\alpha -1/2)}}(x).

in which

(\theta )_{n}

represents the rising factorial of

\theta

One therefore also has the Rodrigues formula

C_{n}^{{(\alpha )}}(x)={\frac {(-2)^{n}}{n!}}{\frac {\Gamma (n+\alpha )\Gamma (n+2\alpha )}{\Gamma (\alpha )\Gamma (2n+2\alpha )}}(1-x^{2})^{{-\alpha +1/2}}{\frac {d^{n}}{dx^{n}}}\left[(1-x^{2})^{{n+\alpha -1/2}}\right].

Orthogonality and normalization

For a fixed α, the polynomials are orthogonal on [−1, 1] with respect to the weighting function (Abramowitz & Stegun p. 774)

w(z)=\left(1-z^{2}\right)^{{\alpha -{\frac {1}{2}}}}.

To wit, for n ≠ m,

\int _{{-1}}^{1}C_{n}^{{(\alpha )}}(x)C_{m}^{{(\alpha )}}(x)(1-x^{2})^{{\alpha -{\frac {1}{2}}}}\,dx=0.

They are normalized by

\int _{{-1}}^{1}\left[C_{n}^{{(\alpha )}}(x)\right]^{2}(1-x^{2})^{{\alpha -{\frac {1}{2}}}}\,dx={\frac {\pi 2^{{1-2\alpha }}\Gamma (n+2\alpha )}{n!(n+\alpha )[\Gamma (\alpha )]^{2}}}.

Applications

The Gegenbauer polynomials appear naturally as extensions of Legendre polynomials in the context of potential theory and harmonic analysis. The Newtonian potential in Rⁿ has the expansion, valid with α = (n − 2)/2,

{\frac {1}{|{\mathbf {x}}-{\mathbf {y}}|^{{n-2}}}}=\sum _{{k=0}}^{\infty }{\frac {|{\mathbf {x}}|^{k}}{|{\mathbf {y}}|^{{k+n-2}}}}C_{{n,k}}^{{(\alpha )}}({\mathbf {x}}\cdot {\mathbf {y}}).

When n = 3, this gives the Legendre polynomial expansion of the gravitational potential. Similar expressions are available for the expansion of the Poisson kernel in a ball (Stein & Weiss 1971).

It follows that the quantities $C_{{n,k}}^{{((n-2)/2)}}({\mathbf {x}}\cdot {\mathbf {y}})$ are spherical harmonics, when regarded as a function of x only. They are, in fact, exactly the zonal spherical harmonics, up to a normalizing constant.

Gegenbauer polynomials also appear in the theory of Positive-definite functions.

The Askey–Gasper inequality reads

\sum _{{j=0}}^{n}{\frac {C_{j}^{\alpha }(x)}{{2\alpha +j-1 \choose j}}}\geq 0\qquad (x\geq -1,\,\alpha \geq 1/4).

References

Abramowitz, Milton; Stegun, Irene Ann, eds. (1983) [June 1964]. "Chapter 22". Handbook of Mathematical Functions with Formulas, Graphs, and Mathematical Tables. Applied Mathematics Series. 55 (Ninth reprint with additional corrections of tenth original printing with corrections (December 1972); first ed.). Washington D.C., USA; New York, USA: United States Department of Commerce, National Bureau of Standards; Dover Publications. p. 773. ISBN 0-486-61272-4. LCCN 64-60036. MR 0167642. ISBN 978-0-486-61272-0. LCCN 65-12253.
Bayin, S.S. (2006), Mathematical Methods in Science and Engineering, Wiley , Chapter 5.
Koornwinder, Tom H.; Wong, Roderick S. C.; Koekoek, Roelof; Swarttouw, René F. (2010), "Orthogonal Polynomials", in Olver, Frank W. J.; Lozier, Daniel M.; Boisvert, Ronald F.; Clark, Charles W., NIST Handbook of Mathematical Functions, Cambridge University Press, ISBN 978-0521192255, MR 2723248
Stein, Elias; Weiss, Guido (1971), Introduction to Fourier Analysis on Euclidean Spaces, Princeton, N.J.: Princeton University Press, ISBN 978-0-691-08078-9 .
Suetin, P.K. (2001), "Ultraspherical polynomials", in Hazewinkel, Michiel, Encyclopedia of Mathematics, Springer, ISBN 978-1-55608-010-4 .

This article is issued from Wikipedia - version of the 11/18/2015. The text is available under the Creative Commons Attribution/Share Alike but additional terms may apply for the media files.

Gegenbauer polynomials

Characterizations

Orthogonality and normalization

Applications

See also

References